Sens de variation, produit et composée avec fonction exponentielle
Exercice corrigé - Spécialité maths, première générale
Énoncé
Étudier le sens de variation de la fonction 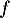 définie par
définie par
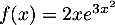 .
.
Préciser l'équation de la tangente au point d'abscisse 0.
Préciser l'équation de la tangente au point d'abscisse 0.
Correction
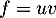 avec
avec 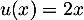 donc
donc 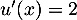 et
et 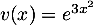 soit
soit  avec
avec
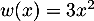 donc
donc 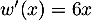 et alors
et alors 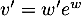 soit
soit 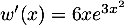 .
.
On a alors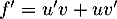 , soit
, soit
![\[f'(x)=2e^{3x^2}+2x\times 6xe^{3x^2}=\left( 12x^2+2\right) e^{3x^2}\]](/Generateur-Devoirs/1S/ChapExp/exvarpc_c/11.png)
On a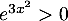 et le premier terme est du second degré de discriminant
et le premier terme est du second degré de discriminant 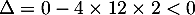 et n'admet donc aucune racine réelle.
et n'admet donc aucune racine réelle.
On a donc
![\[\begin{tabular}{|c|cccc|}\hline
$x$ & $-\infty$ && &$+\infty$ \\\hline
$12x^2+6$ && $+$ &&\\\hline
$e^x$ && $+$ &&\\\hline
$f'(x)$ && $+$ &&\\\hline
&&&&\\
$f$&&\Large{$\nearrow$}&&\\
&&&&\\\hline
\end{tabular}\]](/Generateur-Devoirs/1S/ChapExp/exvarpc_c/14.png)
La tangente a pour équation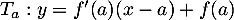 , soit avec
, soit avec  ,
,
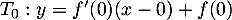 ,
donc ici, avec
,
donc ici, avec 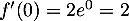 et
et 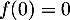 , on trouve l'équation
, on trouve l'équation
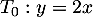
Correction
On aOn a alors
On a
On a donc
![\[\begin{tabular}{|c|cccc|}\hline
$x$ & $-\infty$ && &$+\infty$ \\\hline
$12x^2+6$ && $+$ &&\\\hline
$e^x$ && $+$ &&\\\hline
$f'(x)$ && $+$ &&\\\hline
&&&&\\
$f$&&\Large{$\nearrow$}&&\\
&&&&\\\hline
\end{tabular}\]](/Generateur-Devoirs/1S/ChapExp/exvarpc_c/14.png)
La tangente a pour équation
Tag:Exponentielle
Voir aussi:
Quelques devoirs
Probabilités conditionnelles et calculs de probabilités avec un arbre de probabilités. Propriétés algébriques de l'exponentielle et une étude de fonctionDevoir: Géométrie avec le produit scalaire, et probabilités conditionnelles et arbres de probabilités
étude de fonctions avec exponentielle, calculs de dérivées, et position relative d'une courbe et d'une droite
étude de fonctions avec exponentielle, premier devoir sur les suites: calcul des premiers termes et sens de variation, construction des premiers termes d'une suite
dérivée et sens de variation d'une fonction rationnelle - Calculs algébriques et équations avec exponentielles - Probabilités conditionnelles et arbre de probabilité
équations et inéquations avec exponentielles, variation de fonctions avec exponentielle, probabilités conditionnelle et arbre de probabilité
