Devoir de maths corrigé, Fonctions, suites numériques et une variable aléatoire
Première générale, spécialité mathématiques
Devoir de mathématiques, et corrigé, sur les fonctions, exponentielle, suites numériques et variable aléatoire, posé en spé maths, première générale, année scolaire 2023/2024
Exercice 1: Calculs de dérivées de fonctions
Calculer la fonction dérivée des fonctions 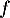 ,
, 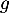 et
et 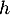 définies par les expressions suivantes:
définies par les expressions suivantes:
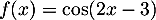 ,
, 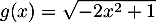 et
et 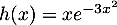
On a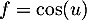 avec
avec 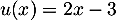 donc
donc 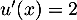
et alors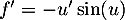 soit
soit 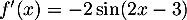
On a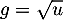 avec
avec 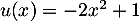 donc
donc 
et alors soit
soit 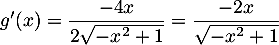
On a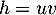 avec
avec 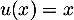 donc
donc 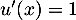 et
et 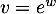 avec
avec 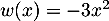 donc
donc 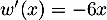 , et donc
, et donc
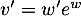 soit
soit 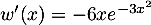
En dérivant le produit, on a alors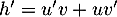 soit
soit
![\[h'(x)=1e^{-3x^2}+x\lp-6xe^{-3x^2}\right)
=\lp1-6x^2\right) e^{-3x^2}
\]](/Generateur-Devoirs/1S/Chap3/exCd2_c/20.png)
Cacher la correction
Correction exercice 1
On a
et alors
On a
et alors
On a
En dérivant le produit, on a alors
Cacher la correction
Exercice 2: Courbe représentative d'une fonction périodique
On considère la fonction 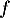 , périodique de période 2, et définie par
, périodique de période 2, et définie par 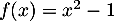 si
si ![$x\in[-1;1]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique/3.png) .
.
Tracer la représentation graphique de la fonction sur
sur ![$[-5;5]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique/5.png)
Sur![$[-1;1]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/1.png) la courbe de
la courbe de  est une portion de parabole. On reproduit ensuite cette portion de parabole par translation sur les intervalles voisins
est une portion de parabole. On reproduit ensuite cette portion de parabole par translation sur les intervalles voisins ![$[1;3]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/3.png) ,
, ![$[3;5]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/4.png) et
et ![$[-3;-1]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/5.png) et
et ![$[-5;-3]$](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/6.png) .
.
![\[\psset{unit=1.5cm,arrowsize=8pt}\begin{pspicture}(-6,-1.8)(6,1)
\psline{->}(-5.6,0)(5.6,0)
\psline{->}(0,-1.6)(0,.8)
\newcommand{\f}[1]{#1 2 exp 1 sub}
% Et son tracer:
\psplot[linewidth=1.4pt]{-1}{1}{\f{x}}
\psplot[linewidth=1.4pt]{1}{3}{\f{x 2 sub}}
\psplot[linewidth=1.4pt]{3}{5}{\f{x 4 sub}}
\psplot[linewidth=1.4pt]{-3}{-1}{\f{x 2 add}}
\psplot[linewidth=1.4pt]{-5}{-3}{\f{x 4 add}}
\multido{\i=-5+1}{11}{\rput(\i,.2){\i}\psline(\i,-.08)(\i,.05)}
\psline(-.1,-1)(.1,-1)\rput(-.3,-1.1){$-1$}
\end{pspicture}\]](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/7.png)
Cacher la correction
Tracer la représentation graphique de la fonction
Correction exercice 2
Sur
![\[\psset{unit=1.5cm,arrowsize=8pt}\begin{pspicture}(-6,-1.8)(6,1)
\psline{->}(-5.6,0)(5.6,0)
\psline{->}(0,-1.6)(0,.8)
\newcommand{\f}[1]{#1 2 exp 1 sub}
% Et son tracer:
\psplot[linewidth=1.4pt]{-1}{1}{\f{x}}
\psplot[linewidth=1.4pt]{1}{3}{\f{x 2 sub}}
\psplot[linewidth=1.4pt]{3}{5}{\f{x 4 sub}}
\psplot[linewidth=1.4pt]{-3}{-1}{\f{x 2 add}}
\psplot[linewidth=1.4pt]{-5}{-3}{\f{x 4 add}}
\multido{\i=-5+1}{11}{\rput(\i,.2){\i}\psline(\i,-.08)(\i,.05)}
\psline(-.1,-1)(.1,-1)\rput(-.3,-1.1){$-1$}
\end{pspicture}\]](/Generateur-Devoirs/1S/Chap3/exCfperiodique_c/7.png)
Cacher la correction
Exercice 3: Variation d'une fonction composée avec exponentielle
Étudier le sens de variation de la fonction 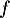 définie sur
définie sur 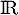 par
par
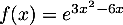 .
.
On a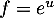 avec
avec 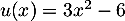 donc
donc 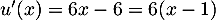 et donc
et donc
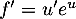 , soit
, soit
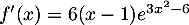
On peut alors dresser le tableau de variation:
![\[\begin{tabular}{|c|ccccc|}\hline
$x$ & $-\infty$ && 1 && $+\infty$\\\hline
$6(x-1)$ && $-$ &\zb&$+$&\\\hline
$e^{3x^2-6}$ && $+$ &$|$ & $+$ &\\\hline
$f'(x)$ && $-$ &\zb&$+$&\\\hline
&&&&&\\
$f$&&\Large{$\searrow$}&&\Large{$\nearrow$}&\\
&&&$e^{-3}$&&\\\hline
\end{tabular}\]](/Generateur-Devoirs/1S/ChapExp/exvarpc2_c/6.png)
Cacher la correction
Correction exercice 3
On a
On peut alors dresser le tableau de variation:
![\[\begin{tabular}{|c|ccccc|}\hline
$x$ & $-\infty$ && 1 && $+\infty$\\\hline
$6(x-1)$ && $-$ &\zb&$+$&\\\hline
$e^{3x^2-6}$ && $+$ &$|$ & $+$ &\\\hline
$f'(x)$ && $-$ &\zb&$+$&\\\hline
&&&&&\\
$f$&&\Large{$\searrow$}&&\Large{$\nearrow$}&\\
&&&$e^{-3}$&&\\\hline
\end{tabular}\]](/Generateur-Devoirs/1S/ChapExp/exvarpc2_c/6.png)
Cacher la correction
Exercice 4: Etude d'une suite arithmético-géométrique avec suite auxiliaire géométrique
On considère la suite  définie par son premier terme
définie par son premier terme
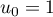 et par la relation, pour tout entier naturel
et par la relation, pour tout entier naturel 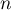 ,
,
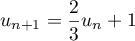 .
.
On considère la suite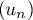 définie par son premier terme
définie par son premier terme
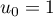 et par la relation, pour tout entier naturel
et par la relation, pour tout entier naturel  ,
,
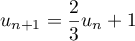 .
.
Cacher la correction
- Calculer
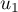 et
et  .
.
- Montrer que
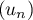 n'est ni arithmétique, ni géométrique.
n'est ni arithmétique, ni géométrique.
- On pose, pour tout entier naturel
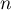 ,
,
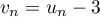 .
.
- Montrer que
 est une suite géométrique,
dont on précisera le premier terme et la raison.
est une suite géométrique,
dont on précisera le premier terme et la raison.
- Exprimer
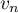 en fonction de
en fonction de 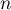 .
.
- En déduire l'expression de
 en fonction de
en fonction de 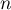 .
.
- Montrer que
Correction exercice 4
On considère la suite
-
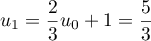 et
et 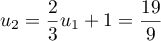 .
.
- On a
 donc
donc  n'est pas arithmétique.
n'est pas arithmétique.
De même, donc
donc  n'est pas géométrique non plus.
n'est pas géométrique non plus.
- On pose, pour tout entier naturel
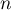 ,
,
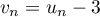 .
.
- Pour tout entier
 ,
,
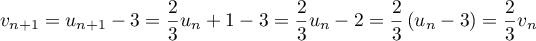 .
.
Ainsi, est une suite géométrique de raison
est une suite géométrique de raison 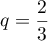 et de premier terme
et de premier terme 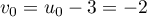 .
.
- On en déduit que, pour tout entier
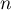 ,
,
 .
.
- On obtient alors,
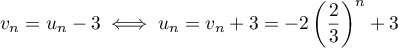 .
.
- Pour tout entier
Cacher la correction
Exercice 5: Espérance et écart type d'une variable aléatoire
On donne la loi de probabilité d'une variable aléatoire 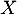 :
:
![\[\begin{tabular}{|*{6}{c|}}\hline
$x_i$ & 12 & 5 & 8 & 9 \\\hline
$P(X=x_i)$ & 0,3 & 0,2 & 0,1 & 0,4 \\\hline
\end{tabular}\]](/Generateur-Devoirs/1S/ChapVA/ex0/2.png)
Calculer l'espérance et l'écart-type de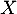 .
(Détailler les formules et calculs effectués.)
.
(Détailler les formules et calculs effectués.)
La moyenne de la série est:
![\[\overline{X}=0,3\tm12+0,2\tm5+0,1\tm8+0,4\tm9 = 9\]](/Generateur-Devoirs/1S/ChapVA/ex0_c/1.png)
La variance de la série est:
![\[
V(X)=0,3\tm(12-9)^2+0,2\tm(5-9)^2+0,1\tm(8-9)^2+0,4\tm(9-9)^2 = 6
\]](/Generateur-Devoirs/1S/ChapVA/ex0_c/2.png)
d'où l'écart-type: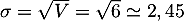
Cacher la correction
Calculer l'espérance et l'écart-type de
Correction exercice 5
La moyenne de la série est:
La variance de la série est:
d'où l'écart-type:
Cacher la correction
Quelques autres devoirs
étude de fonctions avec exponentielle, premier devoir sur les suites: calcul des premiers termes et sens de variation, construction des premiers termes d'une suite
variation d'une fonction composée avec une exponentielle - Deux inéquations avec des exponentielles - Suite numériques explicite et récurrente, construction graphique des premiers termes
Suites: construction graphique des premiers termes, suite intermédiaire arithmétique - Etude d'une suite récurrente avec une suite auxiliaire arithmétique
sur les suites: sommes des termes d'une suite arithmétique et géométrique. Etude d'une suite récurrente avec une suite auxiliaire. Suite récurrente définie avec une fonction exponentielle. Balle rebondissante: hauteur des rebonds et distance totales parcourue
fin d'année, sur les fonctions, exponentielle, suite et variable aléatoire
Quelques exercices corrigés
Exercices corrigés
Construction des premiers termes d'une suite récurrente
Exercices corrigés
Suite récurrente, construction graphique des premiers termes
Exercices corrigés
Suite récurrente avec exponentielle, construction graphique des premiers termes
Exercices corrigés
Sens de variation d'une suite homographique
Exercices corrigés
Calcul des premieres termes et variation d'une suite récurrente
Voir aussi: