Inéquations et tableaux de signe (2)
Exercice corrigé - maths en seconde générale
Énoncé
Résoudre les inéquations:
Correction
![\[\begin{tabular}{|c|ccccccc|}\hline
$x$ & $-\infty$ && $-3$ && $1$ && $+\infty$ \\\hline
$2x-2$ && $-$ &$|$ & $-$ & \mbox{$0\hspace{-0.67em}\mid$} & $+$ & \\\hline
$x+3$ && $-$ &\zb& $+$ & $|$ & $+$ & \\\hline
$(2x-2)(x+3)$ && $+$ &\zb& $-$ &\zb& $+$ & \\\hline
\end{tabular}\]](/Generateur-Devoirs/2nde/Chap3/ex3_c/1.png)
et on lit alors les solutions de l'inéquation
dans la dernière ligne:
![$\mathcal{S}_1=]-\infty;-3]\cup[1;+\infty[$](/Generateur-Devoirs/2nde/Chap3/ex3_c/3.png)
.
Pour l'inéquation 
:
![\[\begin{tabular}{|c|ccccccc|}\hline
$x$ & $-\infty$ && $\frac{5}{3}$ && $3$ && $+\infty$ \\\hline
$-2x+6$ && $+$ &$|$ & $+$ & \mbox{$0\hspace{-0.67em}\mid$} & $-$ & \\\hline
$3x-5$ && $-$ &\zb& $+$ & $|$ & $+$ & \\\hline
$(-2x+6)(3x-5)$ && $-$ &\zb& $+$ &\zb& $-$ & \\\hline
\end{tabular}\]](/Generateur-Devoirs/2nde/Chap3/ex3_c/5.png)
et on lit alors les solutions de l'inéquation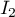
:
![$\mathcal{S}_1=]-\infty;\frac{5}{3}[\cup]3;+\infty[$](/Generateur-Devoirs/2nde/Chap3/ex3_c/7.png)
.
Correction
On dresse le tableau de signe de chaque expression du produit:et on lit alors les solutions de l'inéquation
dans la dernière ligne:
.
:
et on lit alors les solutions de l'inéquation
:
.
Tag:Inéquations et tableaux de signes
Autres sujets au hasard:
Voir aussi: