Intersection d'une parabole et d'une droite
Exercice corrigé - Spécialité maths, première générale
Énoncé
Soit 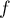 la fonction définie sur
la fonction définie sur 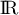 par
par 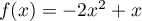 ,
et
,
et 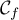 sa courbe représentative dans un repère du plan.
sa courbe représentative dans un repère du plan.
Déterminer les coordonnées des points d'intersection de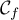 et de la droite d'équation
et de la droite d'équation 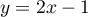 .
.
Déterminer les coordonnées des points d'intersection de
Correction
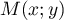 tels que
tels que
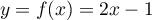 ,
d'où l'équation du second degré
,
d'où l'équation du second degré 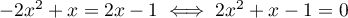 de discriminant
de discriminant 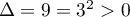 et qui admet donc deux solutions réelles
distinctes
et qui admet donc deux solutions réelles
distinctes 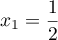 et
et 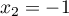 .
.
Il y a donc deux points d'intersection: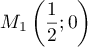 et
et 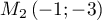 .
.
Correction
Les points d'intersection sont les pointsIl y a donc deux points d'intersection:
Tag:2nd degré
Voir aussi:
Quelques devoirs
équations et inéquations du second degré. Racines d'une fonction trinôme du 2nd degré. Polynome du 3ème degré: factorisation et signe d'une fractoion rationnelle
second degré (équation et inéquation, tableau de signe). Dérivabilité d'une fonction en un point: taux d'accroissement et nombre dérivé (calcul et lecture graphique)
équations et inéquations du second degré. Racines d'une fonction trinôme du 2nd degré
second degré, factorisation d'un polynome du 3ème degré. Équation (réduite) de droite
second degré, factorisation d'un polynome du 3ème degré. Équation (réduite) de droite
