Résolution matricielle d'un système 2x2

Exercice corrigé - Maths expertes, terminale générale

Énoncé

Soit le système 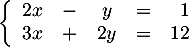$\la\begin{array}{ccccr}2x&-&y&=&1\\3x&+&2y&=&12\enar\right.$
Écrire ce système sous forme matricielle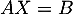 $AX=B$, en précisant les matrices 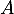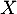$A$, $X$ et $B$.
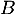Résoudre alors matriciellement ce système.


Correction

Correction

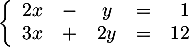Le système $\la\begin{array}{ccccr}2x&-&y&=&1\\3x&+&2y&=&12\enar\right.$ s'écrit sous la forme matricielle 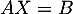$AX=B$, avec les matrices 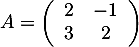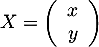$A=\lp\begin{array}{cc}2&-1\\3&2\enar\rp$, $X=\lp\begin{array}{c}x\\y\enar\rp$ et $B=\lp\begin{array}{c}1\\12\enar\rp$.
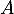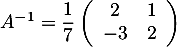
On connaît directement l'inverse de la matrice $A$, soit $A^{-1}=\dfrac17\lp\begin{array}{cc}2&1\\-3&2\enar\rp$
On résout alors le système matriciellement: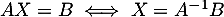 $AX=B\iff X=A^{-1}B$, soit
\[X=\dfrac17\lp\begin{array}{cc}2&1\\-3&2\enar\rp\lp\begin{array}{c}1\\12\enar\rp =\lp\begin{array}{c}2\\3\enar\rp\]



Tag:matrices

Autres sujets au hasard: Lancer de dés


Voir aussi:

Quelques devoirs


LongPage: h2: 3 - h3: 0