Des équations complexes
Exercice corrigé - Maths expertes, terminale générale
Énoncé
Résoudre dans 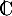 les équations suivantes en donnant les solutions sous forme algébrique:
les équations suivantes en donnant les solutions sous forme algébrique:
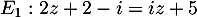
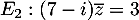
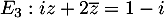
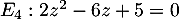
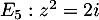
Correction
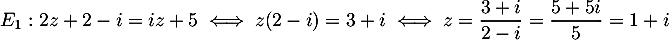
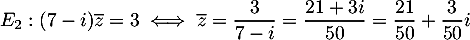
et donc en revenant au conjugué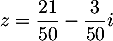
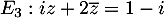 . On pose
. On pose 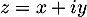 avec des réels
avec des réels 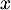 et
et 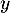 .
.
On obtient alors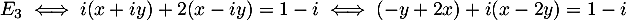 ,
puis en identifiant les parties réelles et imaginaires:
,
puis en identifiant les parties réelles et imaginaires:
![\[\la\begin{array}{lcl}2x-y&=&1\\x-2y&=&-1\enar\right.\]](/Generateur-Devoirs/TS/ChapComplexes/exequations_c/9.png)
et on trouve alors que et
et 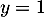 d'où finalement la solution
d'où finalement la solution 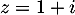
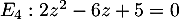 . Cette équation du second degré a pour discriminant
. Cette équation du second degré a pour discriminant
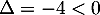 et admet donc deux racines complexes:
et admet donc deux racines complexes:
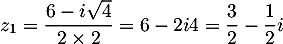 et son conjugué
et son conjugué
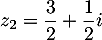 .
.
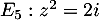 . On pose
. On pose 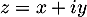 avec des réels
avec des réels 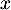 et
et 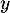 .
.
On obtient alors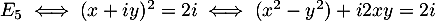 , et alors,
puis en identifiant les parties réelles et imaginaires:
, et alors,
puis en identifiant les parties réelles et imaginaires:
![\[\la\begin{array}{rcl}x^2-y^2&=&0\\xy&=&1\enar\right.\]](/Generateur-Devoirs/TS/ChapComplexes/exequations_c/22.png)
La première équation donne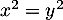 soit
soit 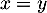 ou
ou 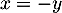 .
.
Si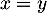 , alors la deuxième équation donne
, alors la deuxième équation donne 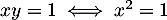 soit
soit  ou
ou 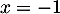 .
.
Si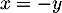 , alors la deuxième équation donne
, alors la deuxième équation donne 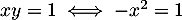 qui est impossible puisque
qui est impossible puisque 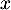 est un nombre réel.
est un nombre réel.
On a donc finalement trouvé deux solutions: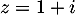 et
et 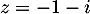 .
.
Correction
et donc en revenant au conjugué
On obtient alors
et on trouve alors que
On obtient alors
La première équation donne
Si
Si
On a donc finalement trouvé deux solutions:
Tag:Nombres Complexes - Algébrique
Voir aussi:
Quelques devoirs
sur les nombres complexes: forme algébrique des nombres complexes et résolution d'équations complexes
sur les nombres complexes: résolution d'équations et forme algébrique. Arithmétique, divisibilité et division euclidienne
sur les nombres complexes, point de vue algébrique. Calculs algébriques sur les nombres complexes, inverse d'un nombre complexe et résolution d'équations
sur les nombres complexes et polynômes. Résolution d'une éuqation complexe. Racine d'un polynôme et factorisation du polynôme. Quotients et restes de division euclidienne. Divisiblité par 8
