Suite d'intégrales et convergence de la série harmonique alternée
Colle de mathématiques
Sujet de colle de maths:Énoncé du sujet
Pour 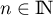 , on définit
, on définit
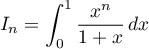 .
.
- Montrer que la suite
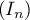 tend vers 0.
tend vers 0.
- Pour
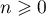 , calculer
, calculer 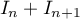 .
.
- En déduire
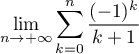
Correction
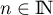 , on définit
, on définit
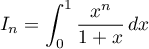 .
.
Correction
Pour- Pour
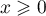 , on a
, on a 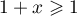 et donc
et donc 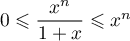 ,
et alors
,
et alors
![\[0\leqslant I_n=\int_0^1\dfrac{x^n}{1+x}\,dx
\leqslant \int_0^1x^n\,dx=\dfrac{1}{n+1}\]](/Generateur-Devoirs/Colles/Integrale/exSI_c/6.png)
On obtient alors, par le théorème des gendarmes,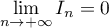 .
.
- Pour
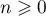 , par linéarité, on a
, par linéarité, on a
![\[\begin{array}{ll}
I_n+I_{n+1}&\dsp=\int_0^1\dfrac{x^n+x^{n+1}}{1+x}\,dx \\[.8em]
&\dsp=\int_0^1 \dfrac{x^n(1+x)}{1+x}\,dx \\[.8em]
&\dsp=\int_0^1 x^n\,dx =\dfrac{1}{n+1}
\enar\]](/Generateur-Devoirs/Colles/Integrale/exSI_c/9.png)
- Soit
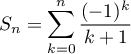 ,
donc d'après ce qui précède,
,
donc d'après ce qui précède,
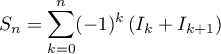 ,
soit en détaillant,
,
soit en détaillant,
![\[
S_n=\left( I_0+I_1\rp-\left( I_1+I_2\rp+\left( I_2+I_3\rp
+\dots+(-1)^n\left( I_n+I_{n+1}\right)
\]](/Generateur-Devoirs/Colles/Integrale/exSI_c/12.png)
et donc, après simplification
![\[S_n=I_0+(-1)^nI_{n+1}\]](/Generateur-Devoirs/Colles/Integrale/exSI_c/13.png)
Comme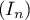 tend vers 0, on en déduit que
tend vers 0, on en déduit que 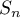 tend vers
tend vers
![$I_0=\dsp\int_0^1\dfrac{1}{1+x}\,dx=\Bigl[\ln(1+x)\Bigr]_0^1=\ln2$](/Generateur-Devoirs/Colles/Integrale/exSI_c/16.png)
Tags:SuitesIntégraleSommes
Autres sujets au hasard:

Voir aussi: