Application linéaire ? Noyau et image ?
Colle de mathématiques
Sujet de colle de maths:- Applications linéairesApplications linéaires
Énoncé du sujet
L'application
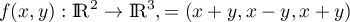 ,
,  .
est-elle linéaire ?
.
est-elle linéaire ?
Préciser son noyau et son image. Est-elle injective ? surjective ? bijective ?
Préciser son noyau et son image. Est-elle injective ? surjective ? bijective ?
Correction
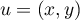 et
et 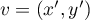 et
et 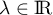 ,
alors
,
alors
![\[\begin{array}{ll}f\lp\lambda u+v\rp&=f\lp(\lambda x+x',\lambda y+y')\rp\\
&=\Bigl( (\lambda x+x')+(\lambda y+y'),(\lambda x+x')-(\lambda y+y'),(\lambda x+x')+(\lambda y+y')\Bigr)\\
&=\Bigl( \lambda(x+y)+(x'+y'),\lambda(x-y)+(x'-y'),\lambda(x+y)+(x'+y')\Bigr)\\
&=\lambda\bigl(x+y,x-y,x+y\bigr)+\bigl(x'+y',x'-y',x'+y'\bigr)\\
&=\lambda f(u)+f(v)\enar\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/4.png)
Ainsi,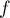 est une application linéaire.
est une application linéaire.
Étudions le noyau de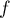 :
:
![\[\begin{array}{ll}(x,y)\in\text{Ker}(f)&\iff f(x,y)=(0,0) \\
&\iff\la\begin{array}{rcl}x+y&=&0\\x-y&=&0\\x+y&=&0\\\enar\right.\\
&\iff\la\begin{array}{rcl}x+y&=&0\\2x&=&0\\\enar\right.\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/7.png)
On en déduit que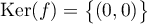 , et, en d'autres termes,
, et, en d'autres termes,
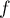 est injective.
est injective.
Étudions maintenant son image:
![\[\begin{array}{ll}
(u,v,w)\in\text{Im}(f)
&\iff\exists (x,y)\in\R^2,\ (u,v,w)=f(x,y) \\[.8em]
&\iff\exists (x,y)\in\R^2,\
\la\begin{array}{rcl}u&=&x+y\\v&=&x-y\\w&=&x+y\enar\right. \\[1.6em]
&\iff\exists (x,y)\in\R^2,\ \la\begin{array}{rcl}u&=&x+y\\u+v&=&2x\\u&=&w\enar\right. \\[2em]
&\iff\exists (x,y)\in\R^2,\ \la\begin{array}{rcl}x&=&\dfrac{u+v}2\\y&=&\dfrac{u-v}2\\u&=&w\enar\right.
\enar\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/10.png)
On en déduit que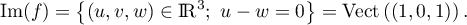
En particulier,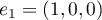 n'est pas dans
n'est pas dans 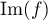 ,
et donc
,
et donc 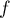 n'est pas surjective, et n'est pas bijective non plus.
n'est pas surjective, et n'est pas bijective non plus.
Correction
Soit![\[\begin{array}{ll}f\lp\lambda u+v\rp&=f\lp(\lambda x+x',\lambda y+y')\rp\\
&=\Bigl( (\lambda x+x')+(\lambda y+y'),(\lambda x+x')-(\lambda y+y'),(\lambda x+x')+(\lambda y+y')\Bigr)\\
&=\Bigl( \lambda(x+y)+(x'+y'),\lambda(x-y)+(x'-y'),\lambda(x+y)+(x'+y')\Bigr)\\
&=\lambda\bigl(x+y,x-y,x+y\bigr)+\bigl(x'+y',x'-y',x'+y'\bigr)\\
&=\lambda f(u)+f(v)\enar\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/4.png)
Ainsi,
Étudions le noyau de
![\[\begin{array}{ll}(x,y)\in\text{Ker}(f)&\iff f(x,y)=(0,0) \\
&\iff\la\begin{array}{rcl}x+y&=&0\\x-y&=&0\\x+y&=&0\\\enar\right.\\
&\iff\la\begin{array}{rcl}x+y&=&0\\2x&=&0\\\enar\right.\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/7.png)
On en déduit que
Étudions maintenant son image:
![\[\begin{array}{ll}
(u,v,w)\in\text{Im}(f)
&\iff\exists (x,y)\in\R^2,\ (u,v,w)=f(x,y) \\[.8em]
&\iff\exists (x,y)\in\R^2,\
\la\begin{array}{rcl}u&=&x+y\\v&=&x-y\\w&=&x+y\enar\right. \\[1.6em]
&\iff\exists (x,y)\in\R^2,\ \la\begin{array}{rcl}u&=&x+y\\u+v&=&2x\\u&=&w\enar\right. \\[2em]
&\iff\exists (x,y)\in\R^2,\ \la\begin{array}{rcl}x&=&\dfrac{u+v}2\\y&=&\dfrac{u-v}2\\u&=&w\enar\right.
\enar\]](/Generateur-Devoirs/Colles/Applin/exAL6_c/10.png)
On en déduit que
En particulier,
Tag:Applications linéaires
Autres sujets au hasard:

Voir aussi: