Égalité des noyaux et images de 3 endomorphismes définis par compositions circulaires
Colle de mathématiques
Sujet de colle de maths:- Applications linéairesApplications linéaires
Énoncé du sujet
Soit 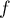 ,
, 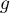 et
et 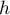 trois endomorphismes d'un même espace vectoriel
trois endomorphismes d'un même espace vectoriel 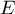 tels que
tels que
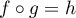 ,
, 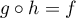 et
et  .
.
Montrer que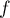 ,
, 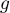 et
et  ont même noyau et même image.
ont même noyau et même image.
Montrer que
Correction
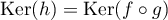 et donc, si
et donc, si  c'est-à-dire
c'est-à-dire 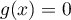 donc
donc  donc
donc  et ainsi
et ainsi
 .
.
De même, en permuttant circulairement, à partir de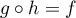 on obtient
on obtient
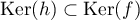 et de
et de 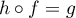 on tire
on tire 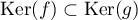 .
.
Finalement, on a obtenu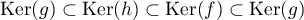 ce qui montre que tous ces noyaux sont égaux.
ce qui montre que tous ces noyaux sont égaux.
Concernant les images, soit par exemple c'est-à-dire qu'il existe
c'est-à-dire qu'il existe  tel que
tel que
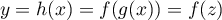 avec
avec  et
donc
et
donc  , d'où
, d'où 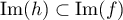
En permuttant à nouveau circulairement,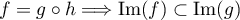 et
et
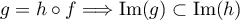 .
.
Finalement, on a obtenu
![\[\text{Im}(h)\subset\text{Im}(f)\subset\text{Im}(g)\subset\text{Im}(h)\]](/Generateur-Devoirs/Colles/Applin/exAL8_c/20.png)
ce qui montre que toutes ces images sont égales.
Correction
De même, en permuttant circulairement, à partir de
Finalement, on a obtenu
Concernant les images, soit par exemple
En permuttant à nouveau circulairement,
Finalement, on a obtenu
ce qui montre que toutes ces images sont égales.
Tag:Applications linéaires
Autres sujets au hasard:

Voir aussi: