Caractérisation d'une composition nulle avec image et noyau
Colle de mathématiques
Sujet de colle de maths:- Applications linéairesApplications linéaires
Énoncé du sujet
Soient 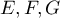 trois
trois 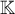 -espaces vectoriels,
et soient
-espaces vectoriels,
et soient 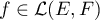 et
et 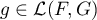 .
.
Démontrer que
![\[g\circ f=0\iff \textrm{Im}f\subset\ker g.\]](/Generateur-Devoirs/Colles/Applin/exAL0/5.png)
Démontrer que
Correction
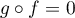 .
.
Soit alors ,
c'est-à-dire qu'il existe
,
c'est-à-dire qu'il existe 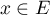 tel que
tel que 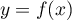 .
.
On a donc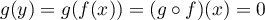 ,
d'où
,
d'où 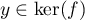 et on a donc obtenu que
et on a donc obtenu que 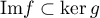 .
.
Réciproquement, supposons que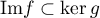 .
.
Soit alors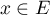 , alors, par définition
, alors, par définition
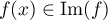 et donc,
par hypothèse
et donc,
par hypothèse 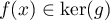 ,
c'est-à-dire
,
c'est-à-dire 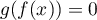 ,
ou encore, ceci étant valable pour tout
,
ou encore, ceci étant valable pour tout 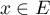 ,
,
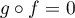 .
.
Correction
SupposonsSoit alors
On a donc
Réciproquement, supposons que
Soit alors
Tag:Applications linéaires
Autres sujets au hasard:

Voir aussi: