Application linéaire ? Noyau et image ?
Colle de mathématiques
Sujet de colle de maths:- Applications linéairesApplications linéaires
Énoncé du sujet
L'application
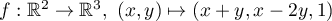 ;
est-elle linéaire ?
;
est-elle linéaire ?
Préciser son noyau et son image. Est-elle injective ? surjective ? bijective ?
Préciser son noyau et son image. Est-elle injective ? surjective ? bijective ?
Correction
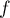 ne peut pas être linéaire car
ne peut pas être linéaire car 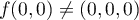 (si elle l'était on devrait en effet avoir
(si elle l'était on devrait en effet avoir
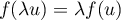 pour tous
pour tous 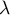 et
et 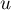 , en particulier donc pour
, en particulier donc pour 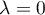 …).
…).
Ceci ne l'empêche pas de pouvoir être éventuellement injective, surjective, bijective.
Le noyau de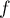 est vide: pour tout
est vide: pour tout 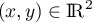 ,
, 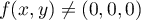 et donc
et donc  .
.
Attention, même si on avait trouvé un noyau réduit au vecteur nul, cela n'aurait pas permis de conclure à l'injectivité de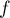 car elle n'est pas linéaire. Il faut revenir à la définition.
Soit deux couples de
car elle n'est pas linéaire. Il faut revenir à la définition.
Soit deux couples de  ,
, 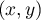 et
et 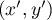 tels que
tels que
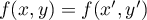 alors
alors
![\[\la\begin{array}{ll}
x+y=x'+y' \\
x-2y=x'-2y'\\
1=1\enar\right.\]](/Generateur-Devoirs/Colles/Applin/exAL2_c/16.png)
soit, en soustrayant les deux premières équations,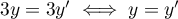 et donc la première équation donne
et donc la première équation donne
 .
.
L'application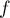 est donc injective.
est donc injective.
Étudions maintenant l'image: soit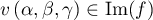 ,
alors il existe
,
alors il existe 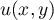 tel que
tel que 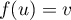 ,
,
![\[\la\begin{array}{ll}
\alpha=x+y\\
\beta=x-2y\\
\gamma=1\enar\right.
\iff
\la\begin{array}{ll}
x=\dfrac{\alpha+\beta}{2}\\
y=\dfrac{\alpha-\beta}{3}\\
\gamma=1\enar\right.
\enar\]](/Generateur-Devoirs/Colles/Applin/exAL2_c/23.png)
Ainsi, tout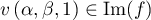 et donc
et donc
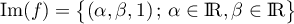 (qui n'est pas un espace vectoriel).
(qui n'est pas un espace vectoriel).
 n'est donc pas surjective, et pas non plus bijective.
n'est donc pas surjective, et pas non plus bijective.
Correction
Ceci ne l'empêche pas de pouvoir être éventuellement injective, surjective, bijective.
Le noyau de
Attention, même si on avait trouvé un noyau réduit au vecteur nul, cela n'aurait pas permis de conclure à l'injectivité de
![\[\la\begin{array}{ll}
x+y=x'+y' \\
x-2y=x'-2y'\\
1=1\enar\right.\]](/Generateur-Devoirs/Colles/Applin/exAL2_c/16.png)
soit, en soustrayant les deux premières équations,
L'application
Étudions maintenant l'image: soit
![\[\la\begin{array}{ll}
\alpha=x+y\\
\beta=x-2y\\
\gamma=1\enar\right.
\iff
\la\begin{array}{ll}
x=\dfrac{\alpha+\beta}{2}\\
y=\dfrac{\alpha-\beta}{3}\\
\gamma=1\enar\right.
\enar\]](/Generateur-Devoirs/Colles/Applin/exAL2_c/23.png)
Ainsi, tout
Tag:Applications linéaires
Autres sujets au hasard:

Voir aussi: