Exercice corrigé - Produit scalaire - Equilibre d'un solide sous l'action de trois forces
Equilibre d'un solide sous l'action de trois forces
Première générale et scientifique
Exercice corrigé de mathématiques: Exercice corrigé - Produit scalaire - Problème complet: Equilibre d'un solide sous l'action de trois forces
Exercice - énoncé:
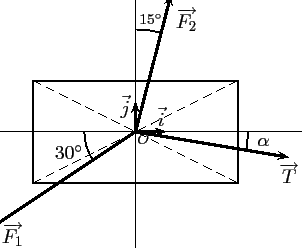
Sur la figure ci-dessous, le rectangle schématise un solide en
équilibre sous l'action des trois forces
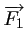 ,
,
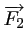 et
et
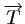 .
.
Cacher la correction
On donne
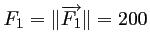 N et
N et
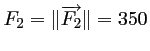 N.
N.
|
|
Correction exercice
- L'équilibre se traduit vectoriellement par la relation:
 .
.
- En projetant cette relation sur
 , on obtient
, on obtient
 ,
,
soit
 ,
d'où,
,
d'où,
 N.
N.
De même, en projetant sur
 , on obtient
, on obtient
 ,
,
soit
 ,
d'où,
,
d'où,
 N.
N.
- L'intensité de
 est alors
est alors
 N.
N.
- On a
 ,
d'où,
,
d'où,
 .
.
Cacher la correction
Voir aussi:
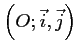 (décompositions des vecteurs sur les deux
orthogonaux du repère), déterminer les composantes
(décompositions des vecteurs sur les deux
orthogonaux du repère), déterminer les composantes 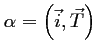 .
.