Quadratic and polynomial forms
Solving quadratic and polynomial equations and inequalities
Contents
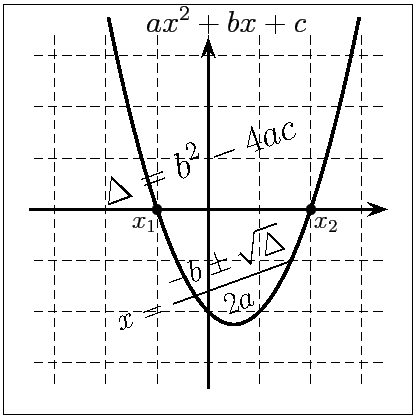
Quadratic form
Solving quadratic equations
Definition
A quadratic expression is an expression which can be written as
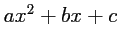 , where
, where
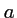 ,
,
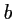 et
et
 represent numbers such that
represent numbers such that
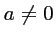 .
.
A number solution of the quadratic equation
solution of the quadratic equation
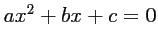 is also called a root of the quadratic
expression
is also called a root of the quadratic
expression 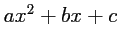 .
.
A number
Example: Some quadratic expressions and their coefficients
| Quadratic expressions |
|
|
|
|
|
|
|
|

|
|
|
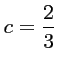
|
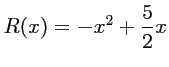
|
|
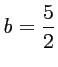
|
|
|
|
|
|
|
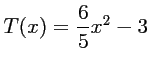
|

|
|
|
|
|
|
|
|
Definition
The number
 is called the discriminant
of the quadratic expression
is called the discriminant
of the quadratic expression
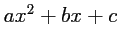
Example: Some quadratic expressions and their discriminant
| Quadratic expressions |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Theorem
 If
If
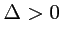 , the quadratic equation
, the quadratic equation
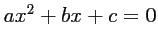 (where
(where 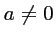 ) has two distinct solutions
(also called roots):
) has two distinct solutions
(also called roots):
 et
et 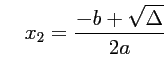
 If
If  , the quadratic equation
, the quadratic equation
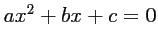 (where
(where 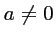 ) has a unique solution
(or root):
) has a unique solution
(or root):

 If
If 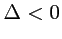 , the quadratic equation
, the quadratic equation
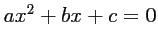 has no real solution.
has no real solution.
Exercice 1. Solve the following quadratic equations
- a)
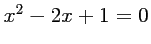
- b)
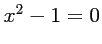
- c)
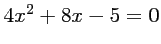
- d)
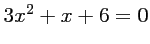
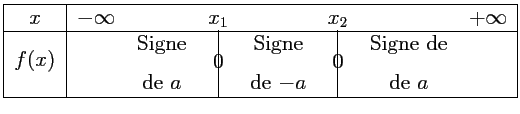
Sign of a quadratic expression
Theorem
Let
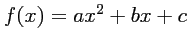 , (
where
, (
where
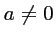 ),
then:
),
then:
 if
if
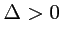 , the equation
, the equation
 has two solutions
has two solutions
 and
and
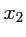 and
and
-
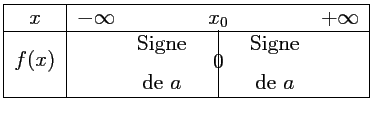
 if
if
 , the equation
, the equation
 has a unique solution
has a unique solution
 and
and
-
 if
if 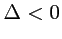 , the quadratic expression
, the quadratic expression
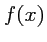 has no root and
has no root and

Exercice 2. Give the sign of the following quadratic functions:
- a)
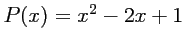
- b)

- c)
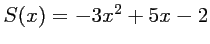
- d)
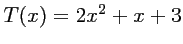
Exercices
Exercice 3. Solve the following inequalities:
- a)
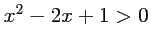
- b)

- c)

Exercice 4. Give the sign of the following expression:
- a)
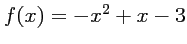
- b)
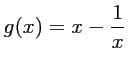
- c)
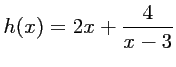
Exercice 5. (Examples of equations reducible to quadratic form)
By first defining
- a)
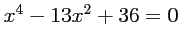
- b)
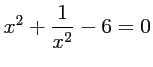
Exercice 6. Determine the intersection points (if they exist) between the parabola
Exercice 7. Determine the intersection points (if they exist) between the two parabolas
Exercice 8. (A parametric quadratic equation)
Let
Determine the values of
Give then this solution.
Polynomial expressions
Fundamental theorem
Definition
A polynomial is an expression which can be written in the form
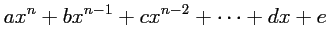 where
where 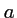 ,
,
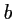 ,
,
 ,
,
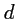 and
and
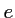 are real numbers, and
are real numbers, and
 is a positive integer.
is a positive integer.
The integer is the degree of the polynomial.
is the degree of the polynomial.
A polynomial is an expression which can be written in the form
The integer
Examples:
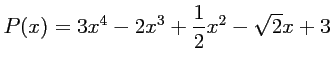 is a polynomial of degree 4.
is a polynomial of degree 4.
Factor Theorem
(Fundamental property for polynomials)
Let be a polynomial of degree
be a polynomial of degree
 and
and
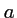 a root of
a root of
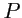 (that is
(that is
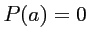 ).
).
Then, can be factored by
can be factored by
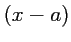 :
there exists a polynomial
:
there exists a polynomial
 of degree
of degree
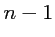 such that
such that
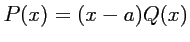
Let
Then,
Exercice 9. We consider the polynomial function
- Show that
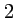 is a root of
is a root of
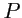 ,
then give a factorization of
,
then give a factorization of
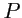 .
.
- Déterminer alors toutes les solutions de l'équation
 .
.
corollary
If the quadratic expression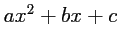 has two roots
has two roots
 and
and
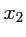 ,
then it can be factored as
,
then it can be factored as
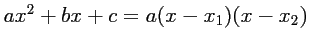 .
.
If the quadratic expression
Exercice 10. Give a factored expression for the following quadratic expressions.
Exercices
Exercice 11. Let the third degree polynomial
- Show that
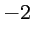 is a root of
is a root of
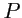 , then give a factored form of
, then give a factored form of
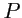 .
.
- Solve the equation
 ,
and then give the sign of the expression
,
and then give the sign of the expression
 .
.
Exercice 12. Beam deflection
A 2 meter length beam is based on three simple supports
The beams supports a uniformly distributed load of 1000 N.m
(8,-0.2)\end{pspicture}$](/Lycee/Common/Cours-2nd-degre/Cours-2nd-degre/img236.png)
One can show that the point located between
- Verify that
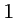 is a solution of this equation.
is a solution of this equation.
- Give a factored form of the polynomial expression of this equation and then solve it.
- Find
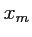 ,
the location between points
,
the location between points
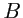 and
and
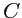 ,
where the beam is at most deformed.
,
where the beam is at most deformed.
Some more exercices on quadratic forms ?